Of all the natural shapes, spirals are considered one of the most common in nature. We find spirals from giant galaxies down to the smallest gastropod shells.
Spirals shape who we are in our DNA double helix and appear in weather patterns as in hurricanes. One spiral giving us incredible potential and the other able to take it all away.
I find spirals to be the most mesmerizing shape of all the natural possibilities. I trace them with my finger on pinecones, gastropod fossils, fern fiddleheads, or even a pineapple at the grocery store. They seem so efficient and compact as well as predictable, all things I appreciate.
Math and natural spirals: Fibonacci numbers
In college, my roommate pointed out my fascination with spirals in nature was based on math equations. I was not excited. She was a math major and I studied wildlife. Unless it was geometry and shapes, math requirements were my nemesis.
She then explained how many of nature’s spirals were based on logarithmic sequencing. She introduced me to Fibonacci numbers as we stared at the center of a sunflower. I was hooked!
Fibonacci was an Italian mathematician. I remember she said scientists believe about 90% of spirals follow Fibonacci numbers.
The Fibonacci sequence of numbers happens like this: each successive number is equal to the sum of the two preceding numbers.
- You start with 1 + 1 = 2.
- Then you take the two preceding numbers to get the sum of the next: 1 + 2 = 3
- And then 2 + 3 = 5
- And so on for 3, 5, 8, 13, 21 etc.
The bracts growing around the base of a pinecone are in a spiral pattern. They can be counted clockwise and counterclockwise. Going clockwise my pinecone has 8 spirals but if I go counterclockwise, I find 13 spirals. Both 8 and 13 are Fibonacci numbers and their sum 21 is the next number in the sequence.
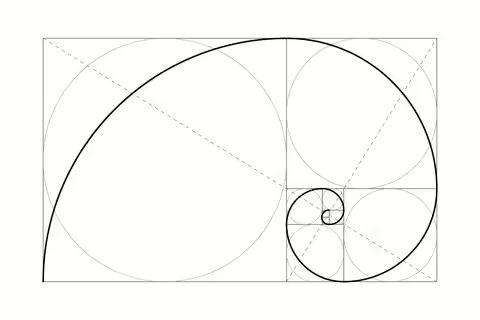
The Golden Ratio
Fibonacci’s numbers are an approximation of what is known as the golden ratio. The golden ratio is 1.61803 and if you start at 21 in the sequence and divide it by the number immediately before it you get a number very close to the golden ratio and will continue to do so as you go forward in the sequence. The larger the numbers in the sequence the more exact it will get. A perfect spiral, one that keeps the same scale with each turn, is considered to follow the golden ratio. A nautilus shell is an example of the golden ratio.
When you look at a plant or animal see if you can find spirals. Count them one way, and if possible, the other and see just how many Fibonacci spirals you encounter. I promise after reading this you will be on a mission that is hard to stop.
MEET THE AUTHOR: Peggy Doty is an energy and environmental stewardship educator who has been with University of Illinois Extension for more than 20 years. She holds a B.S. in zoology from Southern Illinois University and a Master of Educator from Northern Illinois University. She is interested in human and wildlife interactions, supporting native pollinators and water resources.
ABOUT THE BLOG: Naturalist News is a blog by University of Illinois Extension Master Naturalist staff and volunteers who bring you stories highlighting the individuals, places, wildlife and plants that make this state amazing. Join us each week to learn something new, be inspired and become connected to your own community by recognizing the amazing ways we are all intertwined.
Want to get notified when new Naturalist News posts are available? SIGN UP HERE!